What a deviously misleading diagram.
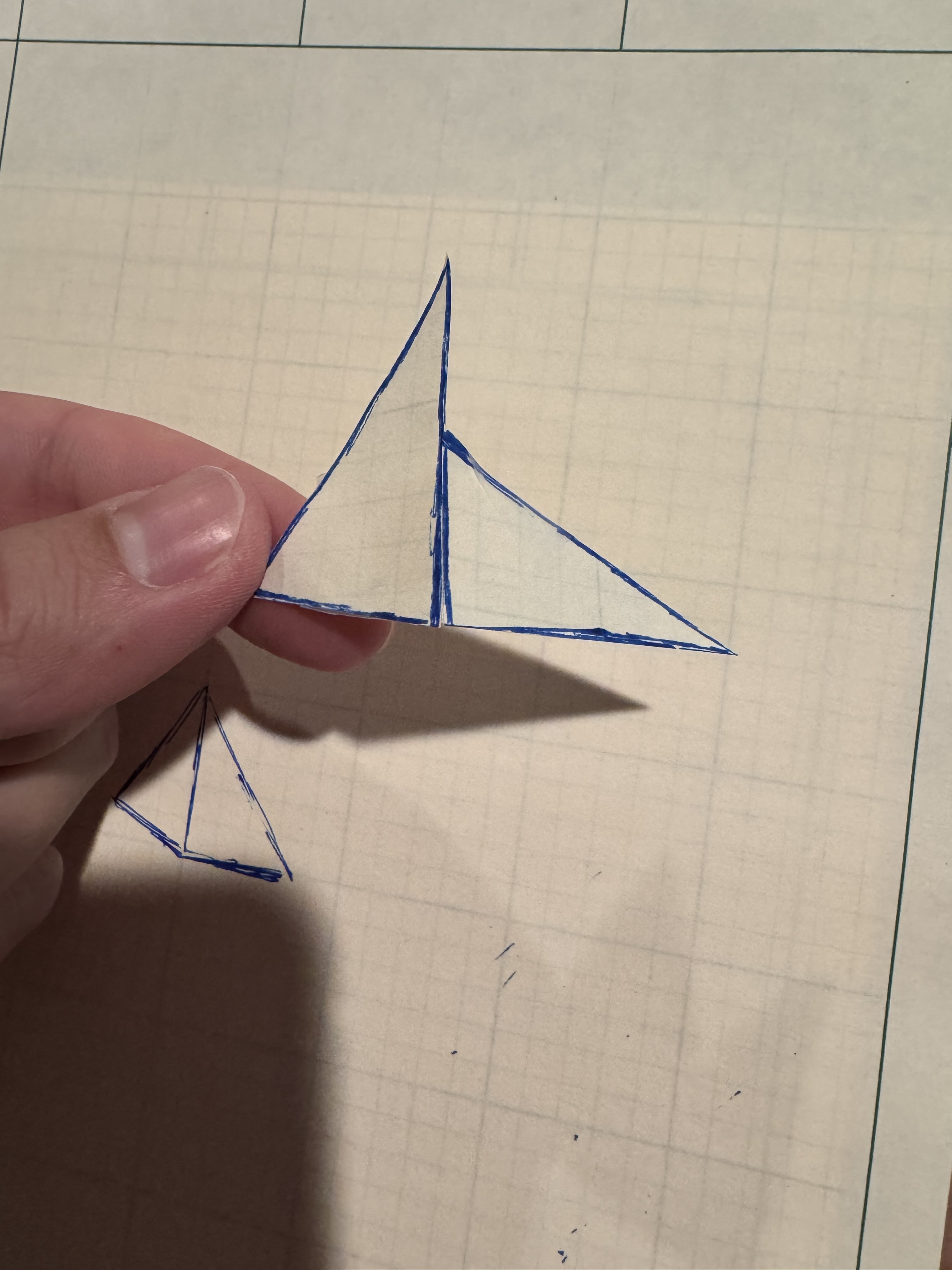
The triangle on the left isn’t actually a right angle triangle, as the other angles add to 100°, meaning the final one is actually 80°, not 90°.
Therefore the triangle on the right also isn’t a right angle triangle. That corner is 100°.
100+35=135°. 180-135=45°. So that’s 45° for the top angle.
X = the straight line of the joined triangles (180°) - the top angle of the right triangle (45°). 180-45=135°
X is 135°, not the 125° it initially appears to be.
It also doesn’t say that the line on the bottom is straight, so we have no idea if that middle vertex adds up to 180 degrees. I would say it is unsolvable.
This is what I was thinking. The image is not to scale, so it is risky to say that the angles at the bottom center add up to 180, despite looking that way. If a presented angle does not represent the real angle, then presented straight lines might not represent real lines.
Eh, I think @sag pretty well nailed it.
Looks like an outer triangle with inner triangles so x = 180 - (180 - (40 + 60 + 35)) = 40 + 60 + 35 = 135
Can you clarify what you mean? this doesn’t make sense to me. There isn’t an “outer” triangle. There’s one triangle (the left one) that has the angles 40, 60, 80. Both triangles are misleadingly drawn as they appear to be aligned at the bottom but they’re not (left triangle’s non-displayed angle is 80, not 90 degrees). So that means we can’t figure out the angles of the right triangle since we only have information of 1 angle (the other can’t be figured out since we can’t assume its actually aligned at the bottom since the graph is now obviously not to scale).
I mean to me it looks like there are two connected triangles with an implied 3rd where x is the degree measure of its apex. IFF that is true, them you can assume 180 degree totals for each triangle individually and one for the “outer triangle”.
I totally get it if you take the perspective that none of it is to scale, but it seems unreasonable to me that a straight line is not a straight line connecting the two triangles shown. Either it’s unsolvable from that premise, or you can assume 3 triangles that compose one larger triangle and solve directly. And it seems weird to share something that is patently unsolvable.
I used to have teacher who deliberately made disproportionate diagrams. His reasoning was that people trust too much what their eyes see and not enough what the numbers tell them. He would’ve loved that diagram.
The person who made it needs this:
I… don’t understand it ?
https://www.explainxkcd.com/wiki/index.php/169:_Words_that_End_in_GRY
I don’t know if that helps or not.
With proper punctuations: There are three words in “the English language”. The other half of it is supposed to be a misdirection.
But yeah, the original joke was really bad in the first place. I don’t blame the second guy for his reaction.
It literally explains it in the comic? People who communicate badly and then act smug when they’re misunderstood are annoying. The other user is saying that the same applies to the OPs post; because the angles don’t match the graphic, they’re communicating badly
Yes but what is communicated badly ? What did the riddle man mean by the three words in english language that end in “gry” ?
The “Riddle Man” wants the answer to be ‘language’ because the question he claims to have asked is what’s the third word in “the English Language.”
If we credulously try to answer the implied question, it’s better to just link this page, but you should read the whole thing.
https://www.merriam-webster.com/help/faq-third-common-gry-word
As the explain xkcd notes, it’s badly told by cueball so it’s impossible to answer. https://www.explainxkcd.com/wiki/index.php/169:_Words_that_End_in_GRY
It’s a geometry puzzle. Of course they aren’t going to get out a protractor to carefully get the 80° drawn to scale. The point of these puzzles isn’t that we actually want to know what the angle is. The point is to navigate a maze of logic. (A very short maze in this particular case.)
Yes, and in this case, the puzzle was poorly presented and likely unsolvable.
Leave it to the Grand Nagus to spot a clever ruse.
12th rule of acquisition : let assumptions work in your favor
I looked at that “90°” angle and went “that doesn’t look right…”
I thought is was wierd that my math didn’t make sense, thanks!
deleted by creator
All these people saying its 135 are making big assumptions that I think is incorrect. There’s one triangle (the left one) that has the angles 40, 60, 80. The 80 degrees is calculated based on the other angles. What’s very important is the fact that these triangles appear to have a shared 90 degree corner, but that is not the case based on what we just calculated. This means the image is not to scale and we must not make any visual assumptions. So that means we can’t figure out the angles of the right triangle since we only have information of 1 angle (the other can’t be figured out since we can’t assume its actually aligned at the bottom since the graph is now obviously not to scale).
Someone correct me if I’m wrong.
135 is correct. Bottom intersection is 80/100, 180-35-100 = 45 for the top of the second triangle. 180 - 45 = 135
Mathematician here; I second this as a valid answer. (It’s what I got as well.)
Random guy who didn’t sleep in middle school here: I also got the same answer.
Random woman who didn’t sleep very well last night. I got a different answer, then thought about it for 10 more seconds and then got 135.
(No I didn’t assume the right angle, my mistake was even dumber. I need a nap.)
You’re making the assumption that the straight line consisting of the bottom edge of both triangles is made of supplementary angles. This is not defined due to the nature of the image not being to scale.
Unless there are lines that are not straight in the image (which would make the calculation of x literally impossible), the third angle of the triangle in the left has to be 80°, making the angle to its right to be 100°, making the angle above it to be 45°, making the angle above it to be 135°. This is basic trigonometry.
which would make the calculation of x literally impossible
Yes.
But that doesn’t mean that line must be straight. It just means if it isn’t, you can’t derive x.
deleted by creator
There is nothing in the image that suggests that the bottom of both triangles forms a straight line.
Except for the part where it’s a single straight line segment, as depicted in the image. Showing the complimentary angles as an unlabeled approximately right angle is within convention. Showing a pair of line segments that do not form a straight line as a straight line is not.
Exactly.
Add to this that
xis literally impossible to calculate if conventions are not assumed, and absolutely possible to calculate if conventions are followed. Assuming the conventions won’t hold is an irrational position.
What you say makes no sense.
The problem is LITERALLY unsolvable if we can’t assume that all the lines are straight.
The schematic was OF COURSE purposefully drawn in a way to make the viewer assume that the third angle of the left triangle is 90°, making the angle to it’s right also be 90°, but the point of the exercise is to get the student to use ALL the given information instead of presuming right angles.
And NO, assuming all the lines are straight is NOT unreasonable, it is the only way that the problem could ever possibly have a solution.
I’d say that the shape on the left has what appears to be a little kink right near X, so one might infer that the shape on the left might be a quadrilateral. There are blatantly obvious vertices that are not labeled as such, so we can’t assume that the not-quite-straight line is supposed to be straight since other angles are also not explicitly indicated as vertices…
deleted by creator
Reasonable assumptions are a fundamental requirement for communication. It’s not that you are wrong in what you are saying. There is a chance that the poser of the question made a visual representation of the triangle’s sides appear to be complementary and appear to construct a straight line across their bases while not actually definitively indicating them as such.
The way these triangle’s are represented is already skewed so perhaps that is what they are trying to do.
The thing is though, at that point they are defying convention and reasonable assumptions so much that they aren’t worth engaging seriously because it’s flawed communication.
The version people are choosing to answer seriously is equivalent to a guy holding up a sign that says “ask me about my wiener to get one in a flash for free!” while standing next to a hot dog stand. If you ask he flashes his junk at you and says cheekily “haha you just assumed wrong! Idiot!”
That’s already dumb enough but some people could see the clues that suggest he was actually intended to flash people the whole time through a series of reasonable assumptions about his outfit lacking pants or the hit dog stand not even being turned on.
Your argument that we can’t assume the line at the bottom is straight is like saying we can’t assume the theoretical trenchcoat man won’t toss a rabid dachshund he was hiding under the coat at us because the hot dog stand has no buns or condiments on it.
You might not be provably wrong but it’s really not worth thinking like an insane person just because a few conventions were defied
So why do you assume the diagram depicts Euclidian space? Why do you assume the numbers are base 10? Why do you assume the question is in English, not just some language that looks like English but has different semantics?
deleted by creator
Following your logic, there is no evidence that these are triangles and it is never stated, therefore none of these lines might be straight and the discussion is irrelevant.
There is also no evidence that these are lines at all and not just unconnected points that are offset on a subpixel scale. Or indeed there is no evidence that they are using base 10 numbers or aren’t asking a completely different question in an invented language that just happens to look like English but has totally different semantics.
The people claiming it is unsolvable because one 110/80 degree pair of angles looks like a 90/90 one are ridiculous.
When you’re finding the outside angle along the line of a triangle you don’t need the inside angle tied to that outside angle if you have the other two inside angles since both straight lines and triangles total to 180 degrees.
This is a standard way to draw geometric proofs, it’s not at all unreasonable to assume straight lines alongside unrepresentative angles. It’s certainly still an assumption, but a conventional one.
I mean, the assumption shouldn’t be anything about scale. It should be that we’re looking at straight lines. And if we can’t assume that, then what are we even doing.
But, assuming straight lines, given straight lines you find the other side of an intersecting line because of complements.
And if we can’t assume that, then what are we even doing
That’s exactly what the other user is saying. We can’t assume straight lines because the given angles don’t make any sense and thus this graph is literally impossible to make. We’re arguing over literal click bait is what we’re doing.
Why do the labeled angles prevent us from assuming straight lines?
Because the angles aren’t represented accurately. It could be that the two angles that look like they’re 90° add up to 180°, but they could also not
That’s technically possible, but that’s also an irrational take. The rational take is to assume the problem is solvable given the available information, which means assuming that the lines are straight.
Yes, two angles appear to be 90⁰, but they’re obviously not with the given information. Math conventions nearly always label right angles, so not having the right angle there implies that the angle should not be assumed to be 90⁰. Math conventions in trigonometry also generally assume straight lines unless there’s a visual indicator that they’re not, and those tend to be exaggerated so it’s obvious.
So the rational answer here is that the bottom line is straight and therefore the problem is solvable. Saying otherwise is irrational, because that’s so far away from math conventions.
Because the apparently straight lines contradict the labels. As drawn, the unlabeled bottom vertices are clearly 90°, not 80° and 100°. We must either conclude that the labels are incorrect, or that the figure is not drawn to scale. Either way, it’s insoluble.
We can’t assume that the straight line across the bottom is a straight line because the angles in the drawing are not to scale. Who’s to say that the “right angle” of the right side triangle isn’t 144°?
If the scale is not consistent with euclidian planar geometry, one could argue that the scale is consistent within itself, thus the right triangle’s “right angle” might also be 80°, which is not a supplement to the known 80° angle.
thx for the compliment
I’d argue that the bottom line is indeed one continuous line regardless of how many other lines intersect on it, because there’s nothing indicating that the line is broken at the intersection.
Now the only reason I think the lines are straight at all is use of the angular notations at the ends, which would be horribly misleading to put at the end of curves or broken lines.
Stupid stuff like this is why kids hate math class. Unless the problem says calculate all unmarked angles, those visually 90 degree angles are 90 degrees. It works that way in any non engineering job that uses angles because it’s common sense.
…what? I get that this drawing is very dysfunctional, but are you going to argue that a triangle within a plane can have a sum of angles of 190°?
Nope I’m not saying that. I’m saying this is a gotcha question that demotivates learners.
I see. I agree completely. The only place this belongs is as a thought experiment on making assumptions in geometry.
The sum of the angles of a triangle are always 180°
Yes, I believe I implied this by suggesting that the sum of angles being 190° is absurd.
No, they’re saying that unless you’re already good at this stuff, it’s easy to assume that a visually 90° angle is actually 90° even when it’s not
Especially if you are bad at this stuff you know that drawing anything like that accurately is a real pain and nobody who can avoid it will ever do it to represent anything accurately. That is what labels are for.
You’re making the assumption that they are triangles.
Your assumption is that it’s a Cartesian coordinate system with 90° angles. But that’s not necessarily the case. You can apply a sheer transformation to correct for the unusual appearance. When you do that, the angles change, but straight lines stay straight and parallels stay parallel. There’s a mathematical term for that, which I can’t remember right now.
135
It pisses me off to no end that what is CLEARLY shown as a 90degree angle is not in fact 90deg, I hate it when they do that.
Also I will sadly admit this can teach people lessons about verifying the information themselves.
GrumbleGrumbleGrumble…
Geometry diagrams in math problems should never be assumed to be to scale
Yes obviously. But it still irritates me as someone who does geometry for a living.
I get you, but it doesn’t clearly indicate the angle in the middle at the base as much as it suggestively waggles its eyebrows towards 90⁰, it could just as easily be 89.9999999999999⁰, although upon zooming in, you can see the line does shift one pixel over on its way up. You simply can’t trust any of the angles as 90⁰ unless it’s got the ∟ symbol (that’s the official unicode) or you’ve measured them yourself, and with that one pixel off-set, it’s decidedly not 90⁰. That’s why you have to do the math.
The internal angles of a triangle always add up to 180⁰, therefore the one pixel offset is irrelevant because the unlabelled angle is, despite what the image suggests,
6080⁰.Assuming you’re talking about the triangle on the left, it’s 80⁰:
180 - 60 - 40 = 80. The other two unlabeled angles are 100⁰ and 45⁰ respectively. None of the unlabelled angles are 60⁰.The shape on the left might be a quadrilateral instead of a triangle, with a vertex at the same place as the top vertex of the shape on the right.
Yes I meant 80 lol, thanks
It’s enough to say it’s “CLEARLY” not 90⁰.
That’s just what I said but more into the weeds on the detail.
👍
Another way to look at it is that it is simply a representation of an object. We don’t need to visualize the angles, as the values to the other asks are given. We just need the geometry of the object represented so we can calculate the value of the unlabeled angle. Given that the geometry of the objects is represented as triangles, we can infer that all sides are straight lines, regardless of the type of space the object occupies.
Actually, it might be a 90 degree angle, but the shape on the left might be a quadrilateral instead of a triangle.
Assuming these are straight lines.
Even if they are straight lines, if that’s a 2d projection of something on a non-flat 3d surface, it can also change the way the angles fit together.
If these aren’t straight lines, drag has no idea what the answer is and thinks it might be impossible to tell.
trash diagram too, the 90 degree looking center angle is actually 80 on the left, 100 on the right.
180 - (100 + 35) = y
x = 180 -y
I can’t be assed to do the simple math
135°.
The non-right-angle is downright cheeky.
Oh my god , those jerks. Lol
trash diagram too
A lot of those standardized tests like SAT or GRE like to put those in (or at least they used to) on purpose. It wasn’t that they couldn’t render the diagrams correctly, instead they were checking for people making assumptions with information that wasn’t given. To be somewhat fair I seem to recall a disclaimer that they weren’t necessarily drawn accurately.
Often they also have multiple slightly different versions of the numbers so people don’t cheat by copying their neighbor’s solution but the diagram is the same.
You mean the simple math of 180 - 180 is too much? Or 100 + 35?
yep. I wasn’t paying enough attention
135
I like that all the comments are people discussing the diagram.
Same.
deleted by creator
But it’s not unsolvable, just a misleading drawing…
It’s not unsolvable at all. The answer is x=135°. The triangles simply aren’t drawn to scale; The line between them isn’t a 90° angle, (even though it is drawn that way) because it is not specifically marked as 90° with a square angle mark.
Right one does not depend on the left one. 3rd dimension for the win!
There’s perils in being in 3d
And thinking so much differently.
deleted by creator
C’man there’s totally a 1px shift on the line. You can’t just assume it’s a right angle.
More importantly, there is enough information in the numbers alone that you don’t have to rely on estimating any angles from the drawing.
The answer is 125 degrees but the triangle on the left has 190 degrees in it
Nah, the angle isn’t specified as a right angle. We can’t assume it’s 90° just because it’s drawn that way, because it isn’t drawn to scale.
Left triangle has 180° total. 60+40=100, which means that middle line is actually 80°, not 90. And since the opposite side is the inverse, we know it is 100° on the other side.
100+35=135. We know the right triangle also has 180° total, so to find the top corner we do 180-135=45. So that top corner of the right triangle is 45°, meaning x must be 135° on the opposite side.
I mean, it’s visibly an acute angle wether it’s labelled as such or not.
125°
Edit: Damn I’m getting roasted for getting it wrong. I totally am wrong, but when I’ve been awake for only 5 minutes that’s bound to happen XD
Removed by mod
It’s a trap. The drawing is misleading. If the left triangle already has 60° and 40° then only 80° remains. Meaning there’s no right angle. The vertical line should be leaning to the left slightly. The correct answer is 135°.
Wrong, as the drawing is not representative. The inner lower angle for the right triangle has to be 100°, as such the inner upper angle has to be 45° and the X angle has to be 135°.
Federation in action: 5 different people from 4 different instances correct OP, not knowing the others have done so, because federating the answers takes a minute.
To be fair I wrote the answer, then figured “surely somebody else must’ve written an answer by now”, refreshed, saw two other answers (one 12 seconds old), thought “fuck it” and posted anyway. They’re all written a bit differently so maybe some are easier to understand than others.
Or 135°
Good on you for admitting fault, not deleting the post, and standing strong. I know you probably feel like a total braindead fucking moron right now - and you’d be right to - which may be the only thing you’ve gotten right in your whole life. /s
5/10 show your work next time! /s
Not to scale. Left triangle shows that the centre tee is actually 80/100⁰, not two right angles. So right triangle is 100+35+45, angle x is 135⁰.
This guy angles
He actually doesn’t lol. It’s 135°.
Nah he definitely does angle. Maybe not super well or anything but there’s clearly some angling happening
the answers here assume that the base is a continuous, straight line
given one of the angles on the left triangle is a right angle on the diagram, but 80 if you calculate it, you can’t assume that
This is a standard way to draw geometric proofs, it’s not at all unreasonable to assume straight lines and unrepresentative angles.
normally in a geometric proof, a right angle is a right angle
This was certainly not my experience in high school. An unlabelled angle could never be assumed. Only angles marked with a square could be taken as right angles.
guess you’re right!
Either this is drawn wrong or they broke geometry
The triangles aren’t drawn to scale. The middle line isn’t a 90° angle, because it isn’t specifically marked with a square angle in the corner. Triangles always add up to 180°, so the angle in the left triangle is actually 80°, not 90°.
Doing 5th grade math makes me feel like a fucking genius. Cant believe I figured it out tbh
Seeing the other comments, it might be worth a shot to repost this meme with your math homework thrown in for easy solutions.
Of course, I did the math too before looking.
Unfortunately, nobody can define a true answer without making assumptions, which is a thought process shown to be faulty by the false right angles.













